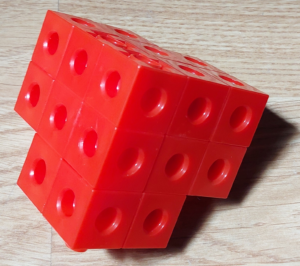
Untitled, plastic cubes and superglue
[15 F, 39 E, 26 V] This shape is the most symmetrical example of a family of polyhedra that can fill all of three-dimensional space by translation, i.e., by only rigidly sliding copies of the shape through space until none of them overlap and there are no gaps. None of the copies need to be rotated at all to reach their final locations, only shifted in position. This shape and the others in its family have analogues as polytopes in any number of dimensions.
The model is made from mass-produced plastic cubes joined together, and fixed with superglue. I made a number of these models in order to understand how they fit together.
Thinking about this class of polytopes led me to discover a new formula for the determinant (see arXiv:2301.06586).