Category: Currently Showing
-

Magic Edges (Truncated cuboctahedron)
Untitled, die-cut printed cardstock. Contributed by Studio Infinity. [26 F, 72 E, 48 V] A cuboctahedron is an Archimedean solid that in some sense is halfway between a cube and a regular octahedron. “Truncating” it means to slice off each of its vertices, creating a new polygonal side beneath where it used to be.
-

Magic Edges (Seventh stellation of the icosahedron)
Untitled, die-cut printed cardstock. Contributed by Studio Infinity. [120 F, 180 E, 62 V] Stellations are created by extending the planes of the faces of a polyhedron until planes from non-adjacent faces meet beyond the original boundaries of the polyhedron. This stellation occupies a position outside the original footprint of the exhibition, illustrating how the…
-

mathgrrl (Pentagonal hexecontahedron)
Pentagonal Hexecontahedron Wireframe, 3D-printed polylactic acid plastic. Contributed by Carolyn Angelillo and Laura Taalman. [60 F, 150 E, 92 V] This model is part of our Catalan wireframe series. The digital model was created using Mathematica, MeshLab, and TopMod, and the physical model was 3D printed on an Ultimaker S3. You can download free files…
-

Yana Mohanty (Campanus’ sphere)
Campanus 2020, reused Tetra paks [72 F, 132 E, 62 V] The globe-like object presented here is called the Campanus’ sphere, after Campanus of Novara, an Italian mathematician, astronomer, astrologer, and physician. The object was actually described by the Euclid in his Elements (c. 300 B.C.E.), but Campanus wrote a popular version of the Elements…
-

Moravian Stars (Triangularly-elevated chamfered icosahedron)
9-inch Tree Topper, molded polyvinyl chloride. Contributed by Studio Infinity. [90 F, 180 E, 92 V] Originating in the 1830s as a geometry project for a boys’ school in Moravia, stars like this one have become a common winter holiday decoration in places spanning the globe. Note that in counting the faces, edges, and vertices…
-

Hiroshi Nakagawa (Rhombicuboctahedron)
Untitled, wood. Contributed by Robert Fathauer. [26 F, 48 E, 24 V] This is an Archimedean solid: all faces are regular polygons, and any vertex can be superimposed on any other vertex by rotating the model, so that all of the faces line up after the rotation.
-

Matt Parker (Regular tetrahedron)
A Sample Tetrahedron, acrylic and zip ties [4 F, 6 E, 4 V] With sides of 3 blue 1 gold it’s the comically small tetrahedron we all didn’t know we needed. Made out of scrap acrylic left over from other projects. Was designed and built from scratch during a swift one-hour livestream on 2023 March…
-

Stepan Paul (Square-elevated triangular prism)
Three Points of Defect, folded paper [14 F, 21 E, 9 V] The “angle defect” of a vertex of a polyhedron is 360 degrees minus the sum of the angles of the faces meeting at that vertex. For example, each vertex of a cube has angle defect 90 degrees, since the three right angles meeting…
-
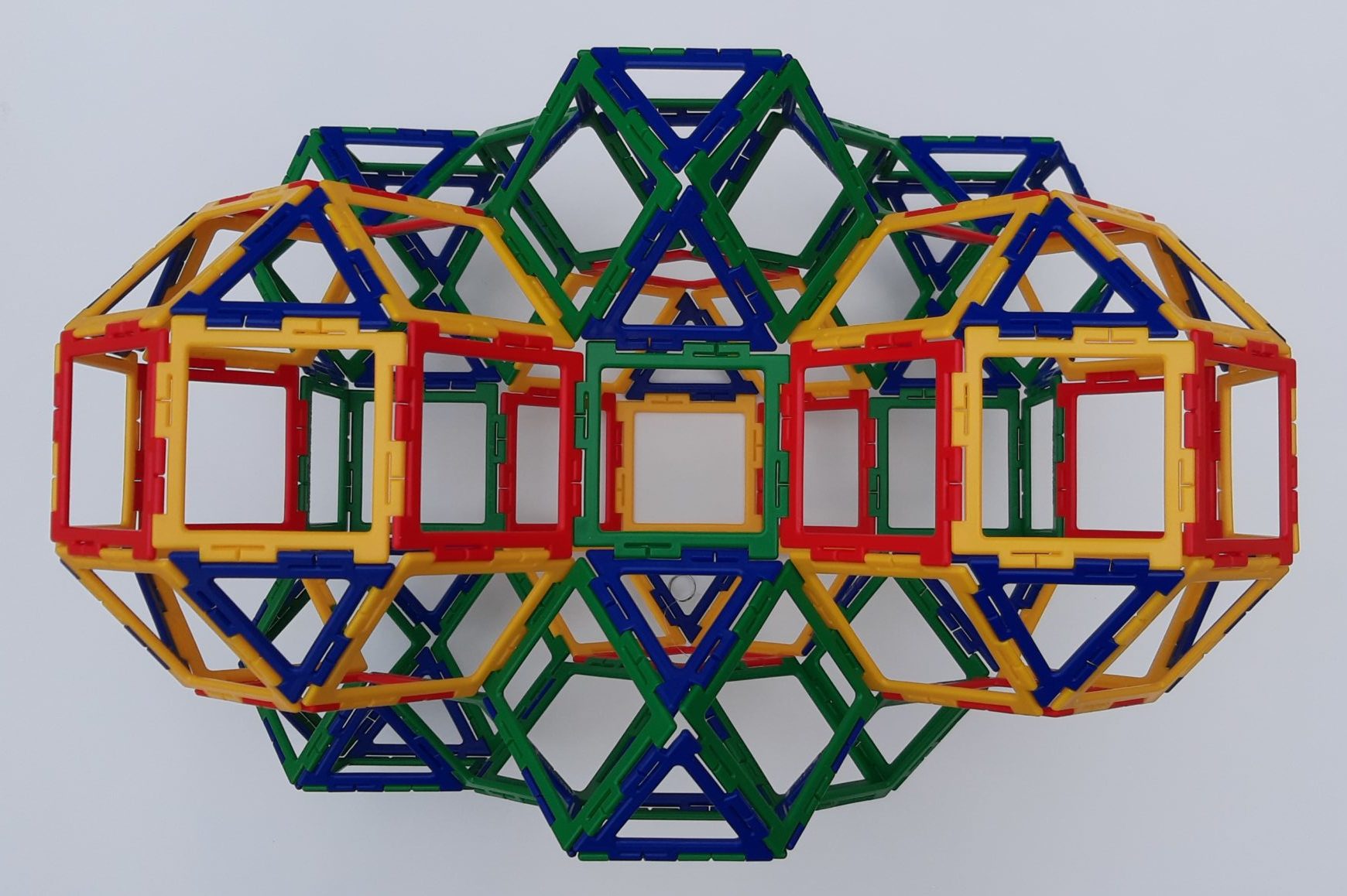
Polydron (Compound of 3 rhombicuboctahedra, 5 cuboctahedra, 6 cubes)
Untitled, injection-molded plastic. Contributed by Studio Infinity. [108 F, 196 E, 90 V] There are many more combinations of symmmetric solids that can fill space with no gaps or overlaps than just the familiar rectangular solids. This construction of Polydron, one of the oldest commercially-produced systems for constructing polyhedra, illustrates one such combination, consisting of…
-

Nick Rauh (Triangulated deltoidal icositetrahedron)
Elevation, plastic drinking straws and embroidery floss [48 F, 72 E, 26 V] This structure starts with a blue tetrahedron and then at each stage elevates it, which is to say, adds pyramids onto each external surface of the previous stage. In this case, the height of each of the pyramids is chosen to make…